There are at least two ways to guarantee investment returns.
The first is to pay off your car loan, then your home loan. Since you’re paying a steady 4% in interest or more, stopping that drain is an immediate 4% return. The biggest push back you’ll hear against my idea applies only to home loans: you can deduct interest expenses when calculating income taxes. However, that is a minor effect. For example, if you’re in a 25% tax bracket, then the return on paying off the loan would be 25% less (3% instead of 4%). However, this is still notable when the return is associated with zero risk.
Another more subtle push back I’ve received is that the mortgage interest may be enough to bring you above the IRS 2% deduction floor that is required to use any deductions, so you can use more deductions. Before going anywhere with the math, remember that the standard deduction is already $12,400 (Married Filing Jointly), so that amount is free. Itemizing deductions is only worth it if you have more than $12,400 in deductions.
Looking more at the numbers reveals that you probably need even more deductions than the standard deduction to make it worthwhile. If your AGI is $80,000 per year, that means you only get to deduct deductions more than $1,600 per year (the 2% floor). Additionally, the benefit of being above the floor should save more than the interest you paid to reach the floor. So let’s assume you have a $100K house loan and you’re paying 4%, or $4,000 in interest each year. That would put you above the 2% floor, however, in order to make back the $4,000 in interest, you’ll need to have deductions 4x as much assuming you’re in the 25% tax bracket. That’s $16,000 in deductions. Do you have that much in deductions above the $1,600 floor?
Paying off loans is one way of guaranteed return. However, there is another more traditional investment that also gives guaranteed return. Zero coupon bonds (ZCB) give a guaranteed dollar amount after a pre-determined length of time. They create some trouble in tax accounting, but that can be alleviated by investing in a ZCB mutual fund.
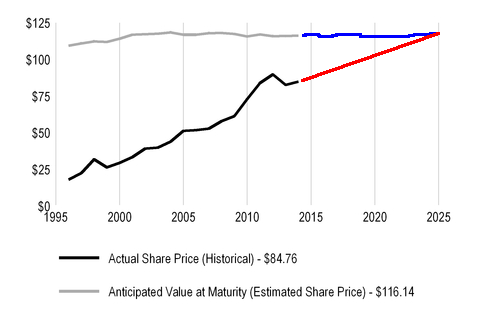
American Century offers three ZCB mutual funds and PIMCO offers a ZCB ETF. I chose BTTRX to demonstrate the numbers below. Page 4 of the BTTRX semi-annual report dated 3/13/14 identifies that the fund share price will be $116.14 in September of 2025. This moves around slightly depending on precisely which ZCB the fund owns at any given time. For example, in September of 2012, the final value was predicted to be $115.81.
We’ll use the most recent estimate of $116.14. The present day price as of November 2014 is $90.50, so the overall return of the fund between now and maturity can be calculated from Rate = 100* [(116.14/PV)^(12/m) – 1], where m is the number of months from now to the end of the fund. Numerically, (116.14 / 90.50) ^ (12/130) = 1.0233, or 2.33% APR.
The graphic below is taken from Page 4 of the BTTRX March 2014 semi-annual report. It shows the actual fund price and the predicted final price for the last 18 years. I added the blue line showing a mostly constant forecast end price. The red line shows predicted share prices, showing the 2.33% profit line, which has to end at the blue line in 2025.
Instead of using the present day price to calculate what the return will be, one can also put in a certain return rate and calculate what price the fund would have to be purchased at to get that return. This can be a simple buy/sell signal telling you when to buy the ZCB. If you buy low and sell high, you’ll get better than the average “buy and hold” rate calculated for the intervening time.
The increasing fund price between now and then forms a sloping straight line on a log axes graph of fund price. Picture this as a rising glideslope to the final known price. One can calculate the price on the line for any time before the final date.
By specifying a 3% glideslope, you can determine what the share price must be at any prior time. If you buy on the glideslope, you’ll have a guaranteed return of 3% averaged over the intervening time.
If the price is higher than the target price, then it’s already accumulated value above the glideslope and shares will earn less than 3% over the remaining time because they must still come out to the same end price. The converse is also true: if the present day price is less than the target, you’ll obtain more than 3% over the remaining life of the fund. Sell above the line, and buy below the line. Can it be much simpler?
The formula to calculate the target price glideslope is PV = 116.14 / 1.03^(m/12). The 1.03 represents the 3%. You can plug in other numbers such as 1.035 for 3.5% or 1.04 for 4% returns. Each return will generate it’s own glideslope approaching the final price. Notice that if you plug 0 (zero) into the formula, then PV comes out equal to the target end value.
Pursuing a 3% return in November 2014, would require a mutual fund price today of PV = 116.14 / 1.03^(130/12) or $84.32. Because the actual price today is a bit higher ($90.50), we would expect to get less than 3% – and this matches our prior calculation of 2.33% APR.
Because we’re in a period of time where the Federal Reserve Bank has suppressed interest rates, current bond prices are therefore higher. Indeed the BTTRX fund has had a bit of a price run-up. When interest rates start to rise again, expect the ZCB fund price to drop – maybe even below the 3% glideslope. That would be a good time to buy more if you want to get better than the 2.33% APR available today.
As a point of comparison, BTTRX has returned 7.23% over the last 10 years. Federal government employees can invest in a Thrift Savings Plan (TSP) “G Fund” that has returned 3.39% over the last 10 years. Recent G Fund returns have been lower: 2.32% over the last 5 years, 1.94% over the last 3 years and 1.89% over the last year. In other words, a steady guaranteed 2.33% seems a pretty good deal these days, and certainly much better than 0.001% available from most money market funds!
In summary, during the next 8+ years, because present day BTTRX prices are high, the price line will drop a little and the rate will come out more than present day 2.33% if you start the investment after the drop. With price fluctuations above and below the average glideslope, you have an opportunity to buy low and sell high because you know where the line is going. This would improve your personalized rate even more. If you want a steady known rate, back-calculate the desired fund price and if the price goes lower than your calculated target, buy the fund at that time and hold until maturity. In the mean time, pay off the car and home loan.
{Update 9/29/2015. BTTRX has 120 months until maturity and it closed yesterday with a price of $95.01. Using the formula given above, we know the future return over the next 10 years will be 100*[(116.14/95.01)^(12/120)-1], or 2.02%. Probably interest rates will rise during the next 10 years and the G fund will do better, so I’m not putting more money into BTTRX until the present price predicts a better return. If rates ~do~ rise, I’m hoping the BBTRX price drops enough to give at least 3-4% return. At that point, I’m buying more shares.}

 99% of ICOs Will Fail
99% of ICOs Will Fail The 10,000 year view of cryptocurrency
The 10,000 year view of cryptocurrency